The effect of plasmid copy number
From 2006.igem.org
(Difference between revisions)
m (Plasmid copy number moved to The effect of plasmid copy number) |
|||
| Line 1: | Line 1: | ||
| - | [[Image: | + | Cell survival in our selection process will require one or more plasmids to be sorted in the correct order and orientation. We used the '''binomial probability distribution''' to model cell survival as a function of number of plasmids and the probability of each plasmid being sorted. This model assumes that the flipping and sorting of each plasmid is independent of the others in the cell. In this model, the probability of cell survival is given by: |
| + | [[Image:dc_binomial.jpg]] | ||
| + | where | ||
| + | * ''n'' is the plasmid copy number | ||
| + | * ''p'' is the probability of a single plasmid being sorted in the correct order and orientation | ||
| + | * ''x'' is the number of plasmids that must be sorted for the cell to survive the antibiotic selection | ||
| - | + | Although we do not know in advance whether a single plasmid being sorted is sufficient for cell survival, we will be able to try different values of ''x'' in the binomial equation, and compare our cell survival rate to the corresponding probabilities. | |
| + | |||
| + | {| | ||
| + | |[[Image:dc_copy_number.jpg]] | ||
| + | |- | ||
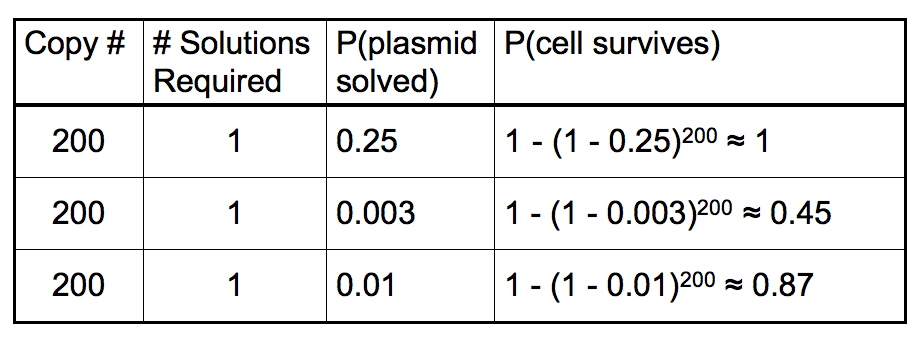
| + | |In the case ''x''=0 (i.e., a single sorted plasmid is sufficient for cell survival), the binomial model simplifies to 1 - (1-p)<sup>n</sup>. In a 2-pancake stack, ''p''=0.25 after several flips. Our binomial model tells us that if the cells have a high-copy number plasmid, and just a single sorted plasmid is sufficient for cell survival, then essentially all of the cells will survive. Therefore, a high-copy number plasmid may make it difficult to verify that flipping is occurring, and at what rate. The effect of a high copy number is less dramatic in the 4-pancake stack, since we should be able to distinguish 0.003 cell survival rate from 0.01 with a low-copy number, as well as distinguish 0.45 cell survival rate from 0.87 with a high-copy number. | ||
Latest revision as of 23:01, 30 October 2006
Cell survival in our selection process will require one or more plasmids to be sorted in the correct order and orientation. We used the binomial probability distribution to model cell survival as a function of number of plasmids and the probability of each plasmid being sorted. This model assumes that the flipping and sorting of each plasmid is independent of the others in the cell. In this model, the probability of cell survival is given by:
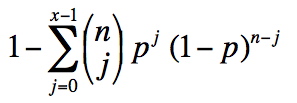 where
where
- n is the plasmid copy number
- p is the probability of a single plasmid being sorted in the correct order and orientation
- x is the number of plasmids that must be sorted for the cell to survive the antibiotic selection
Although we do not know in advance whether a single plasmid being sorted is sufficient for cell survival, we will be able to try different values of x in the binomial equation, and compare our cell survival rate to the corresponding probabilities.