Missouri Western State University 2006
From 2006.igem.org
(→'''Faculty''') |
(→'''Assembly Plan''') |
||
| Line 29: | Line 29: | ||
Shipping Address: Todd Eckdahl, Biology Department, Missouri Western State University, 4525 Downs Drive, Saint Joseph, MO, 64507 [(816) 271-5873] | Shipping Address: Todd Eckdahl, Biology Department, Missouri Western State University, 4525 Downs Drive, Saint Joseph, MO, 64507 [(816) 271-5873] | ||
| + | |||
| + | == '''Project Overview'''== | ||
| + | |||
| + | Solving the Pancake Problem with an E. coli Computer | ||
| + | |||
| + | Our goal is to genetically engineer a biological system that can compute solutions to a puzzle called the burnt pancake problem. The EHOP computer is a proof of concept for computing in vivo, with implications for future data storage devices and transgenic systems. Our work was done in collaboration with the Missouri Western iGEM Team and an undergraduate research fellow from Hampton University. | ||
| + | |||
| + | The Burnt Pancake Problem | ||
| + | The pancake problem is a puzzle in which a scrambled series of units (or stack of pancakes) must be shuffled into the correct order and orientation. You can try solving a simple version of the pancake problem yourself. | ||
| + | |||
| + | |||
| + | Figure 1 A scrambled stack of four burnt pancakes. In the burnt pancake problem, each pancake is given an orientation by burning one side. Figure 1 shows a scrambled stack of burnt pancakes. To solve the problem, every unit, or pancake, must be placed in the proper order (largest on bottom, smallest on top) and in the proper orientation (burnt side down, golden side up). The pancakes are flipped with two spatulas: one to lift pancakes off the top of the stack, the other to flip part (or all) of the remaining stack of pancakes. The pancakes lifted by the first spatula are returned to the top of the stack without being flipped. You can watch Media:burnt_pancake.ogg a movie of the stack in Figure 1 being sorted to see how the puzzle is solved. | ||
| + | |||
| + | |||
| + | Approach | ||
| + | Trial and error is one approach to solving the burnt pancake problem, but how could one compute the quickest solution? Our idea is to let E. coli do the work, using each cell as a tiny processor in a massively parallel machine. A mathematical model of the flipping process helps us design the system and interpret the output of our EHOP computer. | ||
| + | |||
| + | |||
| + | Figure 2 3-D structure of a Hin protein complex bound to DNA. View the interactive Jmol image (PDB 1ZR4).Biological System: The biological representation of a pancake is a functional unit of DNA such as a promoter or coding region. To flip these units of DNA, we have reconstituted the Hin/ Hix invertase system (Figure 2) from Salmonella typhimurium as a BioBrick compatible system in E. coli. Hin invertase (BBa_J31001) was cloned from S. typhimurium, Ames strain TA100 and tagged with LVA. We built the recombinational enhancer (RE) and Hin invertase recognition sequence HixC using the publicly available genomic sequence of S. typhimurium and a dsDNA assembly program we created for gene synthesis from overlapping oligos. | ||
| + | Every segment of DNA flanked by a pair of HixC sites is a "pancake" capable of being inverted. Hin invertase recognizes pairs of HixC sites and inverts the DNA fragment in between the two HixC sites with the help of the Fis protein bound to the RE. In our system, selectable phenotypes (including antibiotic resistance and RFP expression), depend upon the proper arrangement of a series of HixC-flanked DNA segments in a plasmid. This allows us to select for cells that have successfully solved the puzzle. An example of a sorted stack of two pancakes is shown in Figure 3. | ||
| + | |||
| + | |||
| + | Figure 3 A sorted stack of two biological "pancakes" | ||
| + | |||
| + | Simulation results for two pancakes, useful for calibrating kinetics of pancake flipping. Math: Our mathematical model for a stack of pancakes is a signed permutation, in which each numerical value represents the pancake size (or desired position in the stack) and the sign represents the orientation. For example, "1, 2, 3, 4" is a stack of four pancakes all in the proper order and orientation. "-2, 4, -1, 3" is a scrambled stack of the same four pancakes, as shown in Figure 1. Here, pancakes 1 and 2 are in the wrong orientation (burnt side up). A population of E. coli cells (1015 cells, for instance) each carrying ~100 copies of pancake stacks has astounding parallel processing capacity. | ||
| + | Lance, please work on this section. | ||
| + | |||
| + | |||
| + | |||
| + | Methods and Results | ||
| + | Basic parts: Parts used in this project were designed by the Davidson and Missouri Western iGEM teams | ||
| + | Modeling | ||
| + | |||
| + | Modeling the behavior of pancake flipping: deducing kinetics and size biases | ||
| + | Using modeling to choose which families of unsolved pancake stacks to start with | ||
| + | Building the Biological System | ||
| + | |||
| + | Single pancakes | ||
| + | The problems of read-through - uncontrolled Tet expression, uncontrolled flipping | ||
| + | New pSB1A7 vector: insulates, but is not compatible with parts carrying double terminators | ||
| + | Designing pancakes without TT's | ||
| + | Two pancake constructs | ||
| + | Biological equivalence - distinguishing 1,2 from -2,-1 using RFP-RBS, updated panckaes | ||
| + | |||
| + | Conclusions | ||
| + | |||
| + | |||
| + | Consequences of devices: data storage, possible application for rearranging transgenes in vivo, proof-of-concept for bacterial computers, first in vivo controlled flipping of DNA?? | ||
| + | Next steps: can solve problem but need control over kinetics | ||
| + | Lessons learned: | ||
| + | Troubleshooting, communication, teamwork, publicity | ||
| + | Math and Biology meshed really well and even uncovered a new proof | ||
| + | Multiple campuses can increase capacity through communication and cooperation | ||
| + | Size of school is not a limiting factor | ||
| + | We had a blast and learned heaps | ||
| + | |||
| + | -------------------------------------------------------------------------------- | ||
| + | |||
| + | TEAM MEMBERS | ||
| + | |||
| + | Students | ||
| + | |||
| + | Sabriya Rosemond is a junior biology major at Hampton University. | ||
| + | Erin Zwack is a junior biology major at Davidson College. | ||
| + | Lance Harden is a sophomore math major at Davidson College. | ||
| + | Samantha Simpson is a sophomore at Davidson College who might design a major in genomics. | ||
| + | Faculty | ||
| + | |||
| + | A. Malcolm Campbell Department of Biology | ||
| + | Laurie J. Heyer Department of Mathematics | ||
| + | Karmella Haynes Department of Biology - teaching postdoc and visiting assistant professor | ||
| + | |||
| + | -------------------------------------------------------------------------------- | ||
| + | |||
| + | TOOLS AND RESOURCES | ||
| + | |||
| + | iGEM 2006 Jamboree | ||
| + | |||
| + | Presentation Outline | ||
| + | White Board | ||
| + | |||
| + | General Questions | ||
| + | Math Questions | ||
| + | Biology Questions | ||
| + | Biology Tools (Wet Bench) | ||
| + | |||
| + | Pancake Parts | ||
| + | Davidson Protocols | ||
| + | Oligo Cuts Optimization: by Lance Harden | ||
| + | Papers of Interest | ||
| + | Math Tools | ||
| + | |||
| + | Pancake Simulators, aka The Lancelator (MATLAB Code) | ||
| + | Other Flipping Simulators | ||
| + | User-Friendly Version | ||
| + | Power-User Version | ||
| + | Power-User Update | ||
| + | Findmin | ||
| + | Bigtrial | ||
| + | Graphing Tools | ||
| + | Pancakeplotter | ||
| + | Permplotter | ||
| + | Adjmat - creates adjacency matrices | ||
| + | Bioadjmat - Adjmat with biological equivalence | ||
| + | Make_radial - creates a radially-embedded graph | ||
| + | Find_diam - finds the diameter of a pancake graph | ||
| + | Bio-Math Tools | ||
| + | |||
| + | Signedperms - lists all signed permutations for a stack of k pancakes | ||
| + | Bioperms - Signedperms with biological equivalence | ||
| + | Assembly Plans | ||
| + | |||
| + | Assembly powerpoint | ||
| + | Assembly plan | ||
| + | Western Assembly Plan | ||
| + | Davidson Assembly Plan | ||
| + | Progress | ||
| + | |||
| + | Plasmids and Bacteria | ||
| + | Hix and RE | ||
| + | Hin and Antibiotic Pancakes | ||
| + | Ligations | ||
| + | |||
| + | |||
| + | Retrieved from "http://2006.igem.org/Davidson_2006" | ||
| + | |||
== '''Assembly Plan'''== | == '''Assembly Plan'''== | ||
| Line 35: | Line 161: | ||
[[Davidson Assembly Plan]] | [[Davidson Assembly Plan]] | ||
| - | |||
== ''' Math Stuff''' == | == ''' Math Stuff''' == | ||
Revision as of 00:04, 29 October 2006
See [http://www.flickr.com/search/?q=igem+missouri photos] from the Ambassador visit on June 5-6, 2006.
Contents |
Students
• Marian L. Broderick, senior mathematics major, [1]
• Adam D. Brown, senior biology major, [2]
• Trevor L. Butner, senior biochemistry/molecular biology major, [3]
• Lane H. Heard, Central High School (St. Joseph) senior, [4]
• Eric L. Jessen, senior biology major (health sciences), [5]
• Kelly J. Malloy, senior biology/chemistry major (health professions), [6]
• Brad J. Ogden, senior biology major (health sciences), [7]
Faculty
• Todd Eckdahl [http://staff.missouriwestern.edu/~eckdahl/], Professor, Department of Biology, [8]
• Jeff Poet [http://staff.missouriwestern.edu/~poet/], Assistant Professor, Department of Computer Science, Mathematics, and Physics, [9]
Shipping Address: Todd Eckdahl, Biology Department, Missouri Western State University, 4525 Downs Drive, Saint Joseph, MO, 64507 [(816) 271-5873]
Project Overview
Solving the Pancake Problem with an E. coli Computer
Our goal is to genetically engineer a biological system that can compute solutions to a puzzle called the burnt pancake problem. The EHOP computer is a proof of concept for computing in vivo, with implications for future data storage devices and transgenic systems. Our work was done in collaboration with the Missouri Western iGEM Team and an undergraduate research fellow from Hampton University.
The Burnt Pancake Problem The pancake problem is a puzzle in which a scrambled series of units (or stack of pancakes) must be shuffled into the correct order and orientation. You can try solving a simple version of the pancake problem yourself.
Figure 1 A scrambled stack of four burnt pancakes. In the burnt pancake problem, each pancake is given an orientation by burning one side. Figure 1 shows a scrambled stack of burnt pancakes. To solve the problem, every unit, or pancake, must be placed in the proper order (largest on bottom, smallest on top) and in the proper orientation (burnt side down, golden side up). The pancakes are flipped with two spatulas: one to lift pancakes off the top of the stack, the other to flip part (or all) of the remaining stack of pancakes. The pancakes lifted by the first spatula are returned to the top of the stack without being flipped. You can watch Media:burnt_pancake.ogg a movie of the stack in Figure 1 being sorted to see how the puzzle is solved.
Approach
Trial and error is one approach to solving the burnt pancake problem, but how could one compute the quickest solution? Our idea is to let E. coli do the work, using each cell as a tiny processor in a massively parallel machine. A mathematical model of the flipping process helps us design the system and interpret the output of our EHOP computer.
Figure 2 3-D structure of a Hin protein complex bound to DNA. View the interactive Jmol image (PDB 1ZR4).Biological System: The biological representation of a pancake is a functional unit of DNA such as a promoter or coding region. To flip these units of DNA, we have reconstituted the Hin/ Hix invertase system (Figure 2) from Salmonella typhimurium as a BioBrick compatible system in E. coli. Hin invertase (BBa_J31001) was cloned from S. typhimurium, Ames strain TA100 and tagged with LVA. We built the recombinational enhancer (RE) and Hin invertase recognition sequence HixC using the publicly available genomic sequence of S. typhimurium and a dsDNA assembly program we created for gene synthesis from overlapping oligos.
Every segment of DNA flanked by a pair of HixC sites is a "pancake" capable of being inverted. Hin invertase recognizes pairs of HixC sites and inverts the DNA fragment in between the two HixC sites with the help of the Fis protein bound to the RE. In our system, selectable phenotypes (including antibiotic resistance and RFP expression), depend upon the proper arrangement of a series of HixC-flanked DNA segments in a plasmid. This allows us to select for cells that have successfully solved the puzzle. An example of a sorted stack of two pancakes is shown in Figure 3.
Figure 3 A sorted stack of two biological "pancakes"
Simulation results for two pancakes, useful for calibrating kinetics of pancake flipping. Math: Our mathematical model for a stack of pancakes is a signed permutation, in which each numerical value represents the pancake size (or desired position in the stack) and the sign represents the orientation. For example, "1, 2, 3, 4" is a stack of four pancakes all in the proper order and orientation. "-2, 4, -1, 3" is a scrambled stack of the same four pancakes, as shown in Figure 1. Here, pancakes 1 and 2 are in the wrong orientation (burnt side up). A population of E. coli cells (1015 cells, for instance) each carrying ~100 copies of pancake stacks has astounding parallel processing capacity. Lance, please work on this section.
Methods and Results Basic parts: Parts used in this project were designed by the Davidson and Missouri Western iGEM teams Modeling
Modeling the behavior of pancake flipping: deducing kinetics and size biases Using modeling to choose which families of unsolved pancake stacks to start with Building the Biological System
Single pancakes The problems of read-through - uncontrolled Tet expression, uncontrolled flipping New pSB1A7 vector: insulates, but is not compatible with parts carrying double terminators Designing pancakes without TT's Two pancake constructs Biological equivalence - distinguishing 1,2 from -2,-1 using RFP-RBS, updated panckaes
Conclusions
Consequences of devices: data storage, possible application for rearranging transgenes in vivo, proof-of-concept for bacterial computers, first in vivo controlled flipping of DNA??
Next steps: can solve problem but need control over kinetics
Lessons learned:
Troubleshooting, communication, teamwork, publicity
Math and Biology meshed really well and even uncovered a new proof
Multiple campuses can increase capacity through communication and cooperation
Size of school is not a limiting factor
We had a blast and learned heaps
TEAM MEMBERS
Students
Sabriya Rosemond is a junior biology major at Hampton University. Erin Zwack is a junior biology major at Davidson College. Lance Harden is a sophomore math major at Davidson College. Samantha Simpson is a sophomore at Davidson College who might design a major in genomics. Faculty
A. Malcolm Campbell Department of Biology Laurie J. Heyer Department of Mathematics Karmella Haynes Department of Biology - teaching postdoc and visiting assistant professor
TOOLS AND RESOURCES
iGEM 2006 Jamboree
Presentation Outline White Board
General Questions Math Questions Biology Questions Biology Tools (Wet Bench)
Pancake Parts Davidson Protocols Oligo Cuts Optimization: by Lance Harden Papers of Interest Math Tools
Pancake Simulators, aka The Lancelator (MATLAB Code) Other Flipping Simulators User-Friendly Version Power-User Version Power-User Update Findmin Bigtrial Graphing Tools Pancakeplotter Permplotter Adjmat - creates adjacency matrices Bioadjmat - Adjmat with biological equivalence Make_radial - creates a radially-embedded graph Find_diam - finds the diameter of a pancake graph Bio-Math Tools
Signedperms - lists all signed permutations for a stack of k pancakes Bioperms - Signedperms with biological equivalence Assembly Plans
Assembly powerpoint Assembly plan Western Assembly Plan Davidson Assembly Plan Progress
Plasmids and Bacteria Hix and RE Hin and Antibiotic Pancakes Ligations
Retrieved from "http://2006.igem.org/Davidson_2006"
Assembly Plan
Math Stuff
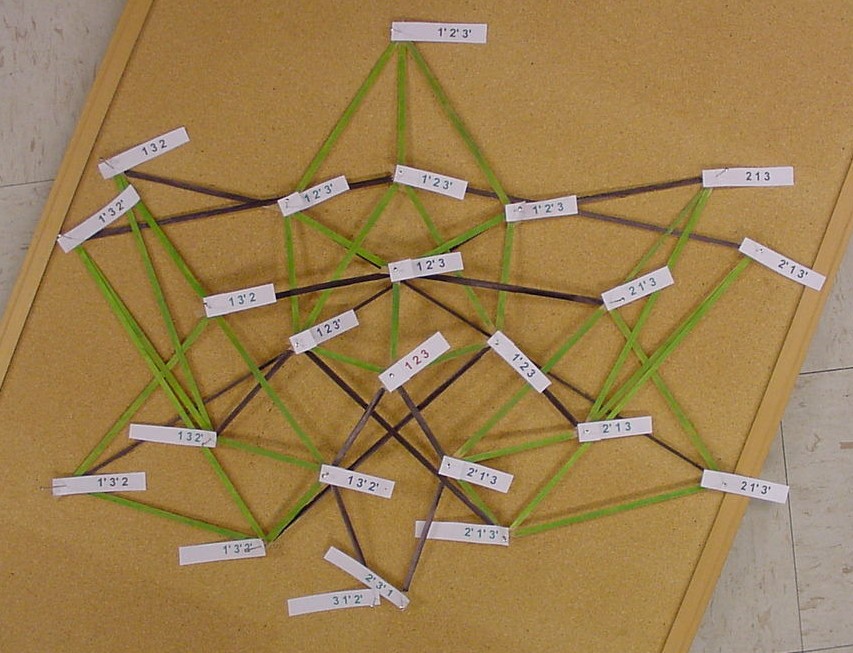
The picture below is of the half graph for the signed permutations for the 3-pancake problem. The full graph would have 48 vertices with each connected to six other vertices. The plan is to transfer this half graph onto the northern hemisphere of a globe with 123 as the north pole, five permutations on the Arctic Circle, eleven on the Tropic of Capricorn, and seven on the equator. An identical graph can be placed on the southern hemisphere such that each permutation occurs diametrically opposite its biological complement. In the picture below, green edges correspond to flips involving one pancake only and black edges correspond to a flip in which a double pancake is involved.
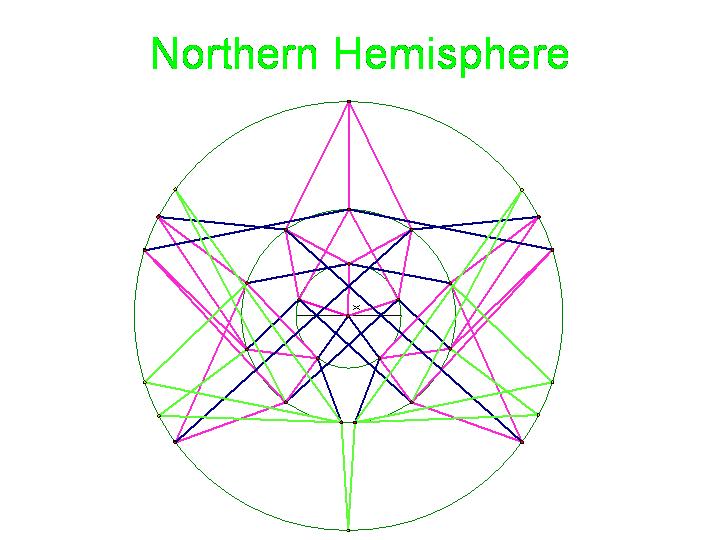
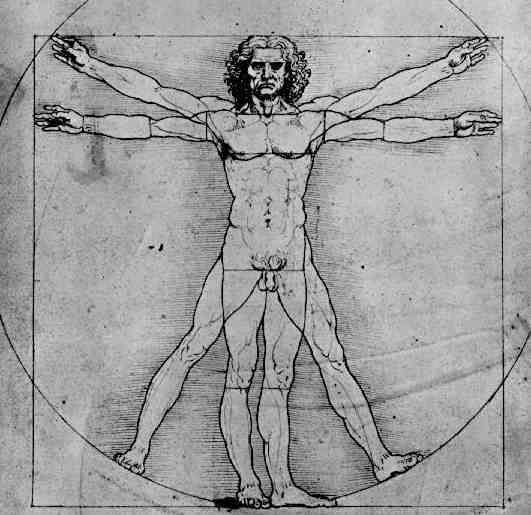
The graphic below was created using Geometer's SketchPad by Marian and shows the 24 vertices on the Northern Hemisphere, similar to the pushpin and rubber band manipulative above. The edges in this graph represent the relationships among the permutations. Pink edges correspond to the flip of a single pancake, black edges to the flip of a double, and green edges to vertices in the Southern Hemisphere. A 3-D model on a globe (okay, it is really a beachball) is in progress. Of curious note, doesn't this graph look similar to Da Vinci's Vitruvian Man?
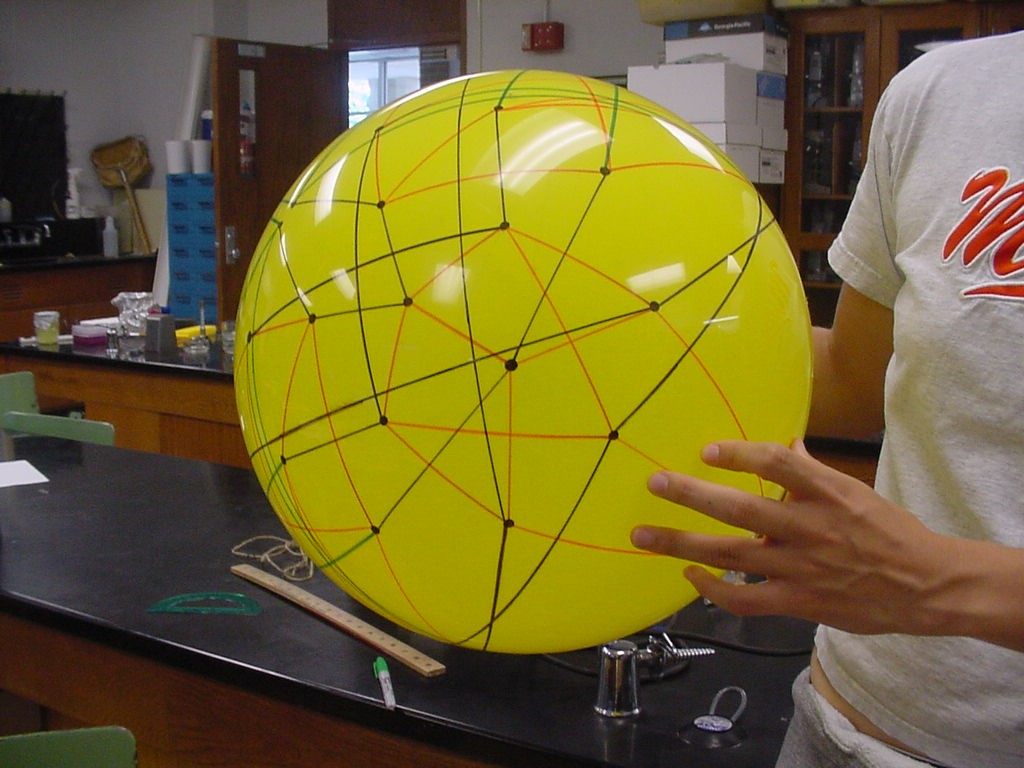
This image is the connected graph of forty-eight vertices on a sphere....finally!
Publicity
MWSU Tower Topics [http://staff.missouriwestern.edu/~eckdahl/iGEM/Tower_Topics_7_2006.htm]
St. Joseph News-Press [http://staff.missouriwestern.edu/~eckdahl/iGEM/St_Joseph_NewsPress_7_2006.htm]
Research Links
OWW Protocols [http://openwetware.org/wiki/Protocols]
Source Plates [http://2006.igem.org/wiki/index.php?title=Biobrick_delivery&printable=yes]
Goodman et al. 1973-1979. The Pancake Problems [http://www.math.uiuc.edu/~west/openp/pancake.html]
Nanassy and Hughes. 1998. In Vivo Identification of Intermediate Stages of the DNA Inversion Reaction Catlyzed by the Salmonella Hin Recombinase [http://www.genetics.org/cgi/content/abstract/149/4/1649]
Lim et al. 1997. Hin-mediated Inversion on Positively Supercoiled DNA [http://www.jbc.org/cgi/content/full/272/29/18434]
Hughes et al. 1992. Sequence-specific interaction of the Salmonella Hin recombinase in both major and minor grooves of DNA [http://www.pubmedcentral.gov/articlerender.fcgi?tool=pubmed&pubmedid=1628628]
Nanassy and Hughes. 2001. Hin Recombinase Mutants Functionally Disrupted in Interactions with Fis [http://jb.asm.org/cgi/content/full/183/1/28?view=long&pmid=11114897]
Lee et al. 1998. In Vivo Assay of Protein-Protein Interactions in Hin-Mediated DNA Inversion [http://jb.asm.org/cgi/content/abstract/180/22/5954]
Merickel et al. 1998. Communication between Hin recombinase and Fis regulatory subunits during coordinate activation of Hin-catalyzed site-specific DNA inversion [http://www.genesdev.org/cgi/content/abstract/12/17/2803]
Haykinson and Johnson. 1993. DNA looping and the helical repeat in vitro and in vivo: effect of HU protein and enhancer location on Hin invertasome assembly [http://www.pubmedcentral.gov/articlerender.fcgi?tool=pubmed&pubmedid=8508775]
Vasquez et al. 2004. Tangle Analysis of Gin Site-specific Recombination [http://math.berkeley.edu/~mariel/Vazquez_Gin04.pdf]
Merickel and Johnson. 2004. Topological analysis of Hin-catalysed DNA recombination in vivo and in vitro [http://www.blackwell-synergy.com/doi/abs/10.1046/j.1365-2958.2003.03890.x]
R.C. Johnson and M.F. Bruist. 1989. Intermediates in Hin-mediated DNA inversion: a role for Fis and the recombinational enhancer in the strand exchange reaction [http://www.pubmedcentral.gov/picrender.fcgi?tool=pmcentrez&blobtype=pdf&artid=400990]
Lim and Simon. 1992. The Role of Negative Supercoiling in Hin-mediated Site-Specific Recombination[http://www.jbc.org/cgi/content/abstract/267/16/11176]
The Pancake Tossing World Record[http://www.recordholders.org/en/records/pancake.html]
Cool site for Breakfast [http://www.cut-the-knot.org/SimpleGames/Flipper.shtml]
iGEM iDeas
Powerpoint with two possible project ideas. [http://staff.missouriwestern.edu/~eckdahl/Research/Synthetic_Biology_2006/IGEMideas%2005-29-06.ppt]
Protocols
To Do List
Since I have severe separation anxiety, post what you did and how things turned out each day right here. Then I can offer suggestions and help plan the next steps.
Monday
Plasmid Prep
Vector Digestions
Develop Assembly Schedule
Tuesday
Trevor: We will have to make reversed RBS and reversed double terminator - design PCR primers for this the way we did for the reversed pAra on Friday and post the proposed sequences here.
cloning hix fragments
Wednesday
Making Reverse pARA
Thursday
Oligos should arrive for reverse RBS and TT (described on Assembly page). Do Reverse Parts with PCR
Pick four colonies off each of the transformation plates (except vector only plates) from yesterday and put into 5 ml LB+Amp at 37C overnight for plasmid preps tomorrow.
Friday
Do plasmid preps on overnight cultures
Measure plasmid yields with UV spec
Digest 200 ng (if that is not most of what we have) of plasmids with XbaI + SpeI and run on 12% PA
Start overnight cultures from transformations done on Thursday
Below are some questions from Jeff and some responses by Todd:
1. Can we double up on some constructions like Promoter(Forward) and Promoter(Reverse) by inserting them into a plasmid that already has a Hix site and getting a 50-50 split (which we can presumably separate out)?
I think it would be better to keep the two promoter orientations as separate constructions.
2. Could we start with a plasmid with a Hix site and insert several things at the same time and then sort them out later? (Example: could we put in a short promoter, a medium length RBS, and a long coding region at the same time and sort them out by length as to which connected? I think this is what you referred to as shotgun ligation when we were discussing the Traveling Salesman Problem.)
Again, I think that keeping things separate will be easier in the long run.
3. Should Hix be the LAST thing in any subconstruction so that we can create a version with HixL, one with HixR, and one with Hix C?
I guess this boils down to the question of how big of a library of parts do we want to create. Is it the case that every time we want to have a Hix site, we need one of each type of Hix? If we take this approach, then we can investigate any differences between the types of Hix sites, perhaps finding the most effective/efficient combination.
The way I see it, there will be four pancakes, each in two orientations, and each one will have a hixC on the right (3') end. That way, each could be used in modular fashion to make any of the four pancake constructs we want.
I think that for now we should head for the construction of parts that can be used in the two different one-pancake devices that will work in the four-pancake ones. I think we should concentrate for now on using hixC only. The problem can be subdivided into the following three devices: Hin recombinase producer, pancake flipping operon, and recombination enhancer.
Davidson Team: We believe we have Hix L, R and C cloned. We need to sequence these to verify. Also, we believe we have RE cloned. Again, we need to sequence this. We are still waiting for PCR primers for Hin. We will tackle this as soon as a pair shows up.
Davidson Team: We like Todd's idea of a part + HixC on the 3' end. The most efficient way to produce this is to use a plasmid with Hix already in it. Do NOT try to cut out a Hix and then clone it. It is too small (26 bp). So always clone the bigger piece into the HixC vector. However, for one pancake devices, we'll need to have Hix on bothsides of Promoter and none on the RBS+coding followed by a separate Terminator.
Davidson Team: We are working on the visuals and will add them to our wiki soon.
From Todd: Great work, Davidson Team! We need to continue to plan our assembly and divide and conquer. Jeff and our students are working on a schedule for assembly and there are also some thoughts at Develop Assembly Schedule. We are working on making reversed pAra this week and will also make plans to make reverse RBS and TT, which we will need eventually.